10. Derivatives and Tangent Lines
a. Derivative at a Point
2. Applications
All the Applications of Limits for rates of change, can now be done using derivatives.
Velocity
If a particle is at position \(x(t)\) as a function of time \(t\), then its velocity at time \(t=a\), is the derivative of the position at \(t=a\), that is \(v(a)=x'(a)\).
Between \(t=0\) and \(t=4\), a girl takes a walk from \(x=0\) to \(x=16\) and back. Her position at time \(t\) is \(x(t)=16-(t-2)^4\). What is her velocity at \(t=3\)?
\(v(3)=x'(3)=-4\)
The velocity is the derivative of \(x(t)\) at \(t=3\). On the \(3^\text{rd}\) line, we cancel the \(16\)'s and expand the numerator. On the \(4^\text{th}\) line we cancel the \(1\)'s in the numerator. On the \(5^\text{th}\) line we cancel the \(h\) from the denominator. \[\begin{aligned} v(3)&=x'(3)=\lim_{h\rightarrow 0} \dfrac{x(3+h)-x(3)}{h} \\ &=\lim_{h\rightarrow 0} \dfrac{[16-(1+h)^4]-[16-(1)^4]}{h} \\ &=\lim_{h\rightarrow 0} \dfrac{-(1+4h+6h^2+4h^3+h^4)+1}{h} \\ &=\lim_{h\rightarrow 0} \dfrac{-(4h+6h^2+4h^3+h^4)}{h} \\ &=\lim_{h\rightarrow 0} -(4+6h+4h^2+h^3)=-4 \\ \end{aligned}\] The velocity is negative because the girl has reversed directions.
Slope
The slope of the tangent line to a function, \(f(x)\), at a point \(x=a\) is the derivative of the function at \(x=a\), that is \(m=f'(a)\).
Find the slope of the tangent line to the function, \(f(x)=6x^2-x^3\), at \(x=3\).
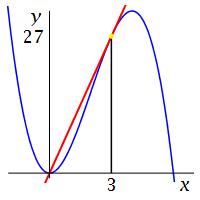
Slope: \(m=f'(3)=9\)
The slope is the derivative of \(f(x)\) at \(x=3\).
On the \(3^\text{rd}\) line, we expand \((3+h)^2\) and \((3+h)^3\).
On the \(4^\text{th}\) line, we cancel and combine terms in the numerator.
On the \(5^\text{th}\) line we cancel the \(54\) in the numerator and then
the \(h\) from the denominator.
\[\begin{aligned}
m&=f'(3)=\lim_{h\rightarrow 0} \dfrac{f(3+h)-f(3)}{h} \\
&=\lim_{h\rightarrow 0} \dfrac{[6(3+h)^2-(3+h)^3]-[6\cdot3^2-3^3]}{h} \\
&=\lim_{h\rightarrow 0} \dfrac{[6(9+6h+h^2)-(27+27h+9h^2+h^3)]-[54-27]}{h} \\
&=\lim_{h\rightarrow 0} \dfrac{54 + 36h + 6h^2 -27 - 27h -9h^2 -h^3 - 27}{h} \\
&=\lim_{h\rightarrow 0} \dfrac{9h-3h^2 -h^3}{h} \\
&=\lim_{h\rightarrow 0} (9-3h-h^2)=9
\end{aligned}\]
Other Rates of Change
The rate of change of any quantity, \(Q(t)\), at time \(t=a\) is the derivative of the quantity at \(t=a\), that is \(Q'(a)\).
The volume of water in a rain gauge at time \(t\) is given by \(V(t)=\sqrt{t}\). What is the rate, \(V'(4)\), at which the gauge is filling at \(t=4\)?
\(V'(4)=\dfrac{1}{4}\)
The rate at which the gauge is filling is the derivative \(V'(4)\).
On the \(2^\text{nd}\) line, we multiply the numerator and denominator
by the conjugate.
On the \(3^\text{rd}\) line, we expand in the numerator using difference
of squares.
On the \(4^\text{th}\) line we simplify the numerator and cancel the
\(h\) from the denominator.
\[\begin{aligned}
V'(4)&=\lim_{h\rightarrow 0} \dfrac{R(4+h)-R(4)}{h}
=\lim_{h\rightarrow 0} \dfrac{\sqrt{4+h}-\sqrt{4}}{h} \\
&=\lim_{h\rightarrow 0} \dfrac{(\sqrt{4+h}-\sqrt{4})}{h}
\dfrac{(\sqrt{4+h}+\sqrt{4})}{(\sqrt{4+h}+\sqrt{4})} \\
&=\lim_{h\rightarrow 0} \dfrac{(4+h)-(4)}{h(\sqrt{4+h}+\sqrt{4})} \\
&=\lim_{h\rightarrow 0} \dfrac{1}{\sqrt{4+h}+\sqrt{4}}=\dfrac{1}{4} \\
\end{aligned}\]
Heading
Placeholder text: Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum Lorem ipsum